The term “factors of 75” refers to the sum of a number multiplied by a different number to create the original numbers. A factor may be described as an algebraic phrase which evenly divides an number with an zero remainder. In other words it’s also referred to for the totality of a number of elements. Some of the factors can be positively or negatively. To learn more about the factors in depth.
Prime factors of 75 by division method calculator
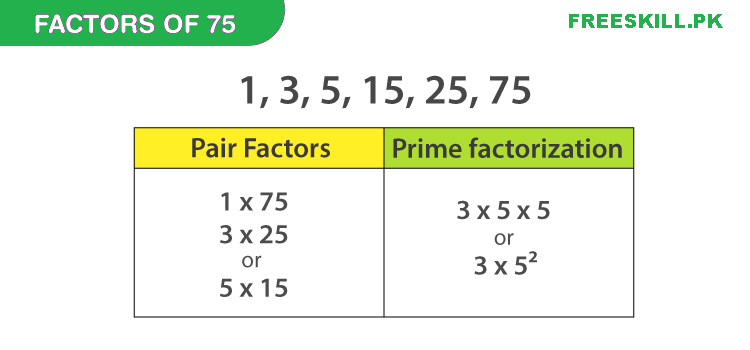
Factors of 75 in Pairs
- In the beginning, we must look at the number that we will need to identify the elements. We are looking for the variables of 75.
- Then we look for those pairs that, when multiplied, give us the number 75.
- So1x75=751×75=75Thus, 1 and 75 are the factors in 75.
- In the same way, we will discover additional reasons.
3×25=75,(3,25).3×25=75,(3,25).
5×15×75,(5,15).5×15×75,(5,15).
25×3×75,(25,3).25×3×75,(25,3).
15×5×75,(15,5).
Pairs
If 1 × 75 = 75, then (1, 75) is a pair factor of 75.
Similarly, let us find another pair.
3 × 25 = 75, (3, 25) is a pair factor of 75
5 × 15 = 75, (5, 15) is a pair factor of 75
25 × 3 = 75, (25, 3) is a pair factor of 75
15 × 5 = 75, (15, 5) is a pair factor of 75
Here,(3, 25) is the same as (25, 3) and (5, 15) is the same as (15, 5)
Division Method To Find the Factors of 75
When using the factorization technique to factorize, we first look at the numbers 1 and 75, as factors that make up 75, then you can find additional pairs of factors that when multiplied give us the original number.
Negative numbers considered factors as when we multiply two negative numbers, we obtain an amount that is positive. there are no changes to the numbers that we’re analyzing the variables.
Another method of calculating the 75th factor is to use the divisibility method.
the factors that make up 75. characteristics of the factors. Steps to discover the pair factors of the year 75 factorization method for determining the factors of 75.
Prime Factorization of 75
This method is where the number 75 first divided by the prime number with the lowest value which is 2. If it doesn’t divide 2 by itself, we move on to the next prime number that is 3.
In this case, 75 could be divided by 3 , so we can divide it. If it’s feasible to divide it by 3, we’ll divide it again by 3. In the event that it is not possible, we will move towards the number that is next in prime.
With this method it is possible to find the percentages of 75.
How to Find the Prime Factors of 75 by Division Method
Follow the steps below to determine the factors of an amount.
- Then, write down the number 75.
- Locate the numbers which will give you 75 when multiplied, such as 3 and 25 like 3 25 x 3 = 75.
- We are aware the prime number which is composed of only two elements, i.e., 1 and the number itself( 3 and 1). This means that it can’t be further digitized.
- 3 = 3 x 1
- However, take a look at the number 25 that can be described as a composite and not an integer prime number. Therefore, it is able to be further to be factorized.
- 25 = 5 x 5 x 1
- Thus, the factorization of 75 is written as = 3 5 x 3 1
- Write down the numbers that are unique (i.e.,) 3 5 x 3 1.
Prime Factors OF 75
The first thing to remember is first that prime numbers comprise any positive integer that can be only equally divided by one and then by itself. Prime Factors of75 are all prime numbers which when multiplied, equal 75.
The prime factors for 75 is 75 = 3x 5 5. Let’s write all the variables from 75 using prime factors.
- First step: Add all numbers together and then multiply just two at the same time. 3, 5, 5
- 2. multiply each one by another number a second time. i.e. 3 x 5 = 15 and 5 x 5 = 25. So, the results are 15, 25, and 25.
- Step 3. Write down all the terms in the figure i.e. 1, 3, 5, 15, 25, 75
Once we’ve done one factorization prime, then we are able to multiply them to obtain additional factors. Are you able to figure whether all the factors are covered? As you may have thought that for the prime number, there aren’t any other variables.
Bunsen Burner Parts and Functions